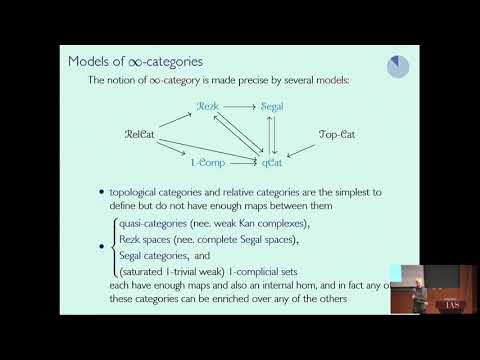
Homotopy type theory provides a “synthetic” framework that is suitable for developing the theory of mathematical objects with natively homotopical content. A famous example is given by (∞,1)-categories — aka “∞-categories” — which are categories given by a collection of objects, a homotopy type of arrows between each pair, and a weak composition law. In this talk we’ll compare two “synthetic” developments of the theory of ∞-categories — the first (joint with Verity) using 2-category theory and the second (joint with Shulman) using a simplicial augmentation of homotopy type theory due to Shulman — by considering in parallel their treatment of the theory of adjunctions between ∞-categories.