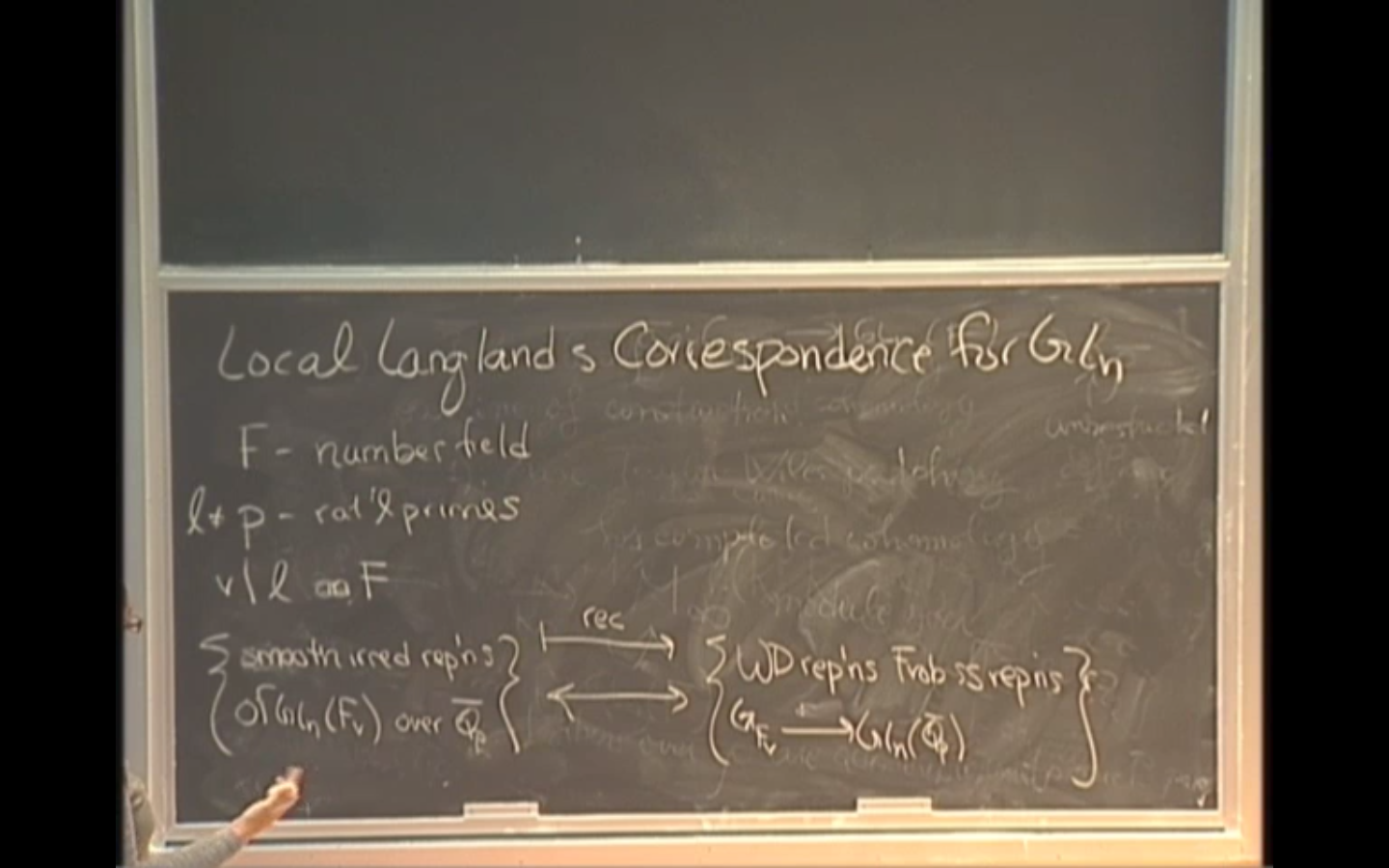We prove the compatibility of local and global Langlands correspondences for GLn up to semisimplification for the Galois representations constructed by Harris-Lan-Taylor-Thorne. More precisely, let r_p(π) denote an n-dimensional p-adic representation of the Galois group of a CM field F attached to a regular algebraic cuspidal automorphic representation π of GL_n(A_F ). We show that the restriction of r_p(π) to the decomposition group of a place v \not |p of F corresponds up to semisimplification to rec(π_v), the image of π_v under the local Langlands correspondence. Furthermore, we can show that the monodromy of the associated Weil-Deligne representation of r_p(π)|Gal_(F_v) is "bounded by" the monodromy of rec(π_v). To prove the above, we use the fact that the representations r_p(π) are constructed as a p-adic limit of representations for which local-global compatibility is already known. We are able to p-adically interpolate the traces of these representations (as well as their exterior powers), which allows us to establish the above results. If time permits, we will discuss how this argument may be modified to study the Galois representations constructed by Scholze at primes away from p.