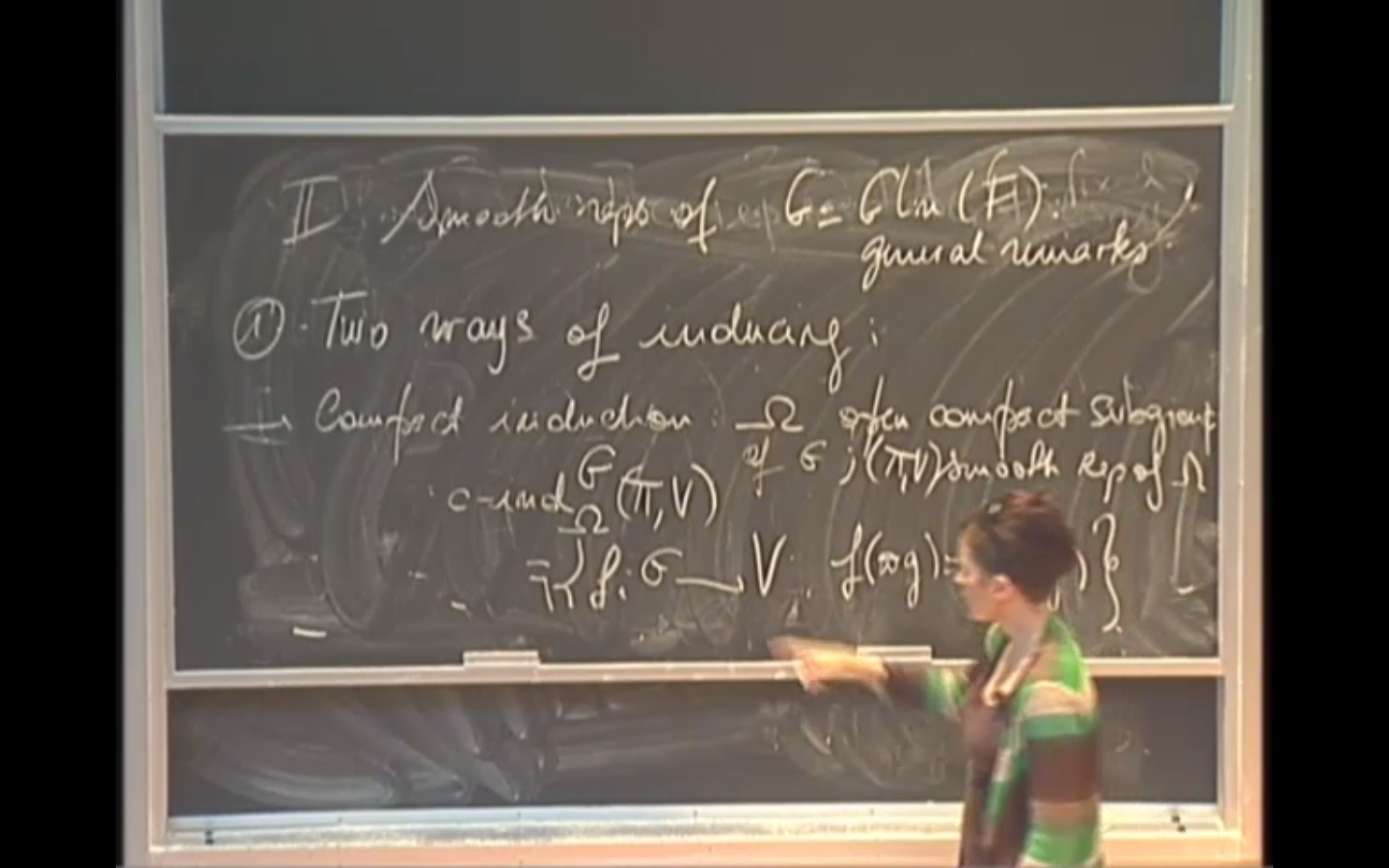These lectures will focus on the mod p representation theory of a split p-adic reductive group G, using GL(2) as a running example. We hope to emphasize the differences between the mod p and complex representations of G while keeping in mind that the theory is partly motivated by the mod p and complex local Langlands programs. We will start with remarks regarding finite reductive groups. We will then compare the homological properties of certain categories of mod p and complex representations of G (and the associated pro-p-Iwahori Hecke algebra). In particular, in the complex setting, the theory of coefficient systems on the Bruhat-Tits building by Schneider and Stuhler gives a way to construct explicit projective resolutions. We will explore what remains from this theory in the mod p setting. This will help us describe the first step in the construction of Colmez' functor yielding the mod p local Langlands correspondence for GL(2,Q_p).