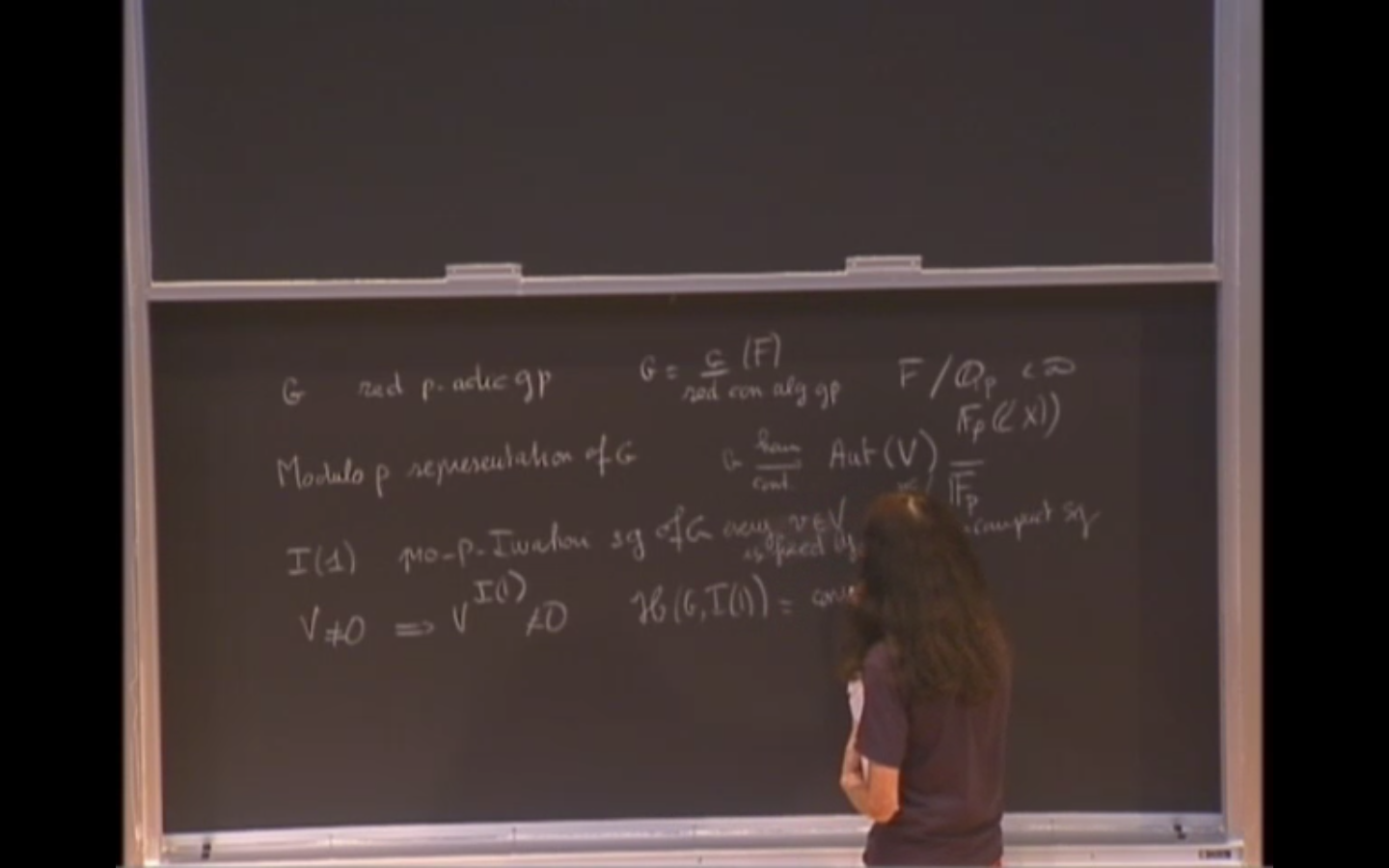Pro-p-Iwahori-Hecke algebras of p-adic groups.
Presenter
August 29, 2014
Keywords:
- pro-p Iwahori-Hecke algebra
- alcove walk
- admissible representation
- parabolic induction
- supercuspidal representations
MSC:
- 14F30
- 22E35
- 33D80
- 51E24
- 20E42
- 20E34
- 20E36
- 20E18
- 20E15
- 20Exx
- 11F33
Abstract
Let (W, S) be a Coxeter system with length ell, R a commutative ring, (q_s, c_s) a family of elements of R, constant on the intersections with S of the conjugacy classes of W. The R-algebra H_R(W, S, q_s, c_s) is the free R-module of basis (T_w)w∈W with product satisfying the relations:
Braid relations: T_w T_w' = T_(ww') for w, w' ∈ W with ell(w) + ell(w') = ell(ww');
Quadratic relations: T_s^2 = q_s +c_s T_s for s in S.
These algebras are variants of the convolution algebra H_R (G, I(1)) of the double cosets of a pro-p-Iwahori subgroup I(1) of a p-adic reductive group G. The algebras H_R(G, I(1)) play a key role in the modulo p representation theory of G via the I(1)-invariant functor. We will describe the alcove walk bases, the Bernstein relations in H_R(G, I(1)), and the simple supersingular modules when R is an algebraically closed field of characteristic p.