Counting Characters of p'-degree
Presenter
March 14, 2008
Keywords:
- Lie theory
- finite groups of Lie type
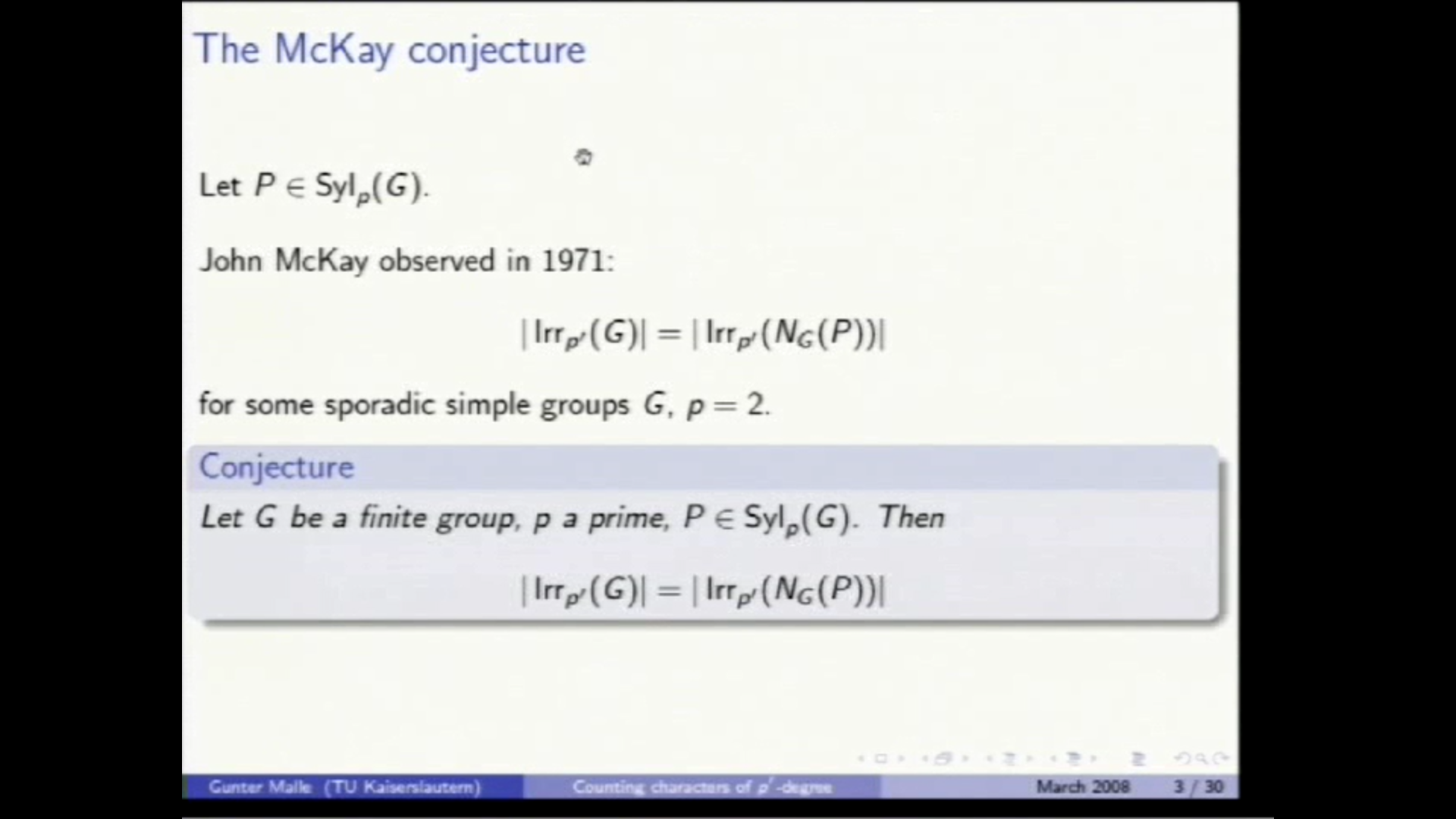
- McKay conjecture
- Sylow subgroups
- character theory
- modular representation theory
MSC:
- 17-xx
- 17Bxx
- 20C33
- 20C40
- 20D05
- 20D06
- 20D15
- 20D20
- 20D30
- 20C15
- 20Cxx
- 20-xx
Abstract
The emphasis will be on the interplay of combinatorics, Lie theory and finite group theory. Connections between these areas go back at least to Schur and Weyl: representations of the symmetric group, polynomial representations of general linear group, Weyl’s character formula. In the last 20 years the field has seen great development as the combinatorics of Young tableaux has been generalized to any Lie type via the theory of crystals. Littlemann’s path model approach to crystals makes a strong connection between this theory and the geometry of the ?ag variety and recent activity is exploring the connection between representation theory, the geometry of the loop Grassmannian and affine Hecke algebras. In the modular representation theory of finite groups of Lie type, connections with complex geometry have arisen in the defining characteristic case and with affine Kac-Moody algebras in the non-defining characteristic case. Topics covered by the workshop will include finite groups of Lie type, algebraic groups, quantum groups, affine Lie algebras, Hecke algebras, cluster algebras, W-algebras, and modular Lie algebras.