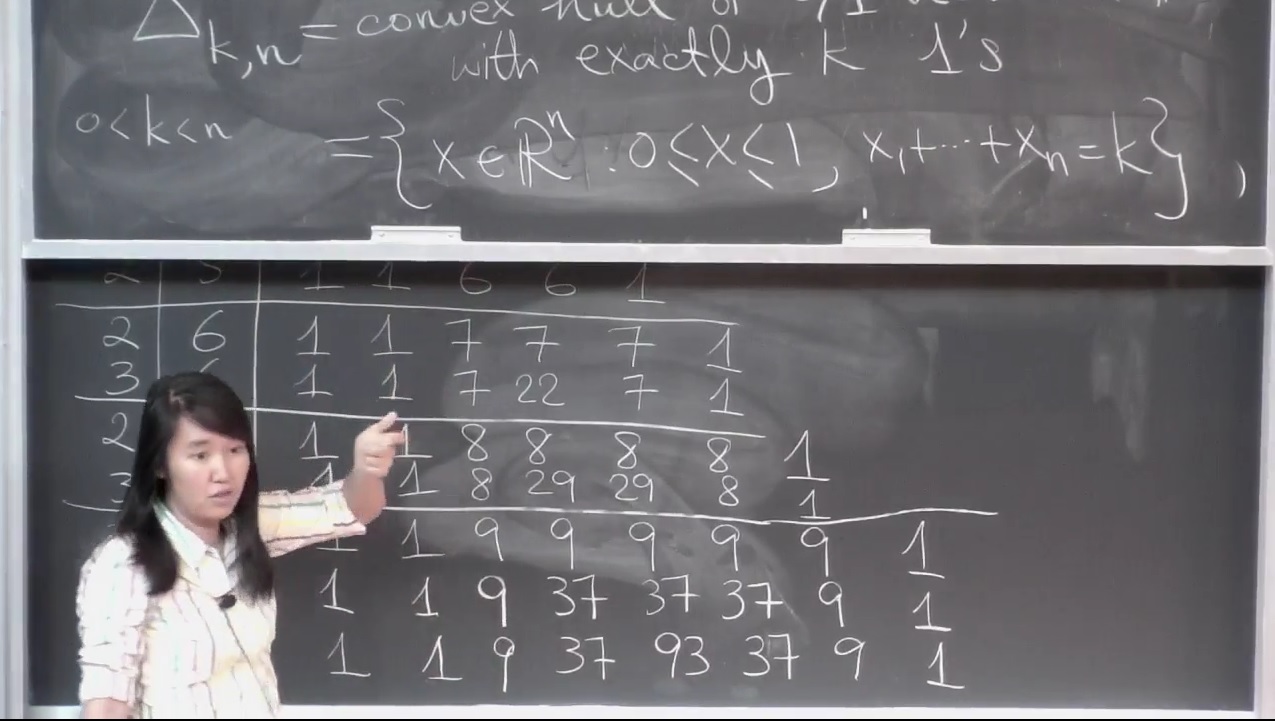The Chow Betti numbers of a hypersimplex are ranks of Chow cohomology groups of the torus orbit closure of a generic point in the Grassmannian. They are also dimensions of Minkowski weights on the normal fan of the hypersimplex, which are functions on the set of cones of the fan satisfying a balancing condition. We give explicit formulas for these numbers. We also show that similar formulas hold for the toric h numbers of dual hypersimplices and coordinator numbers of type $A^*$ lattices. This is based on a joint work with Charles Wang.