Period Polynomial Relations among Double Zeta Values and Various Generalizations
Presenter
March 27, 2017
Keywords:
- Galois theory
- Galois orbits
- periods
- motivic geometry
- algebraic geometry
- Riemann zeta function
- multiple zeta values
- motivic zeta values
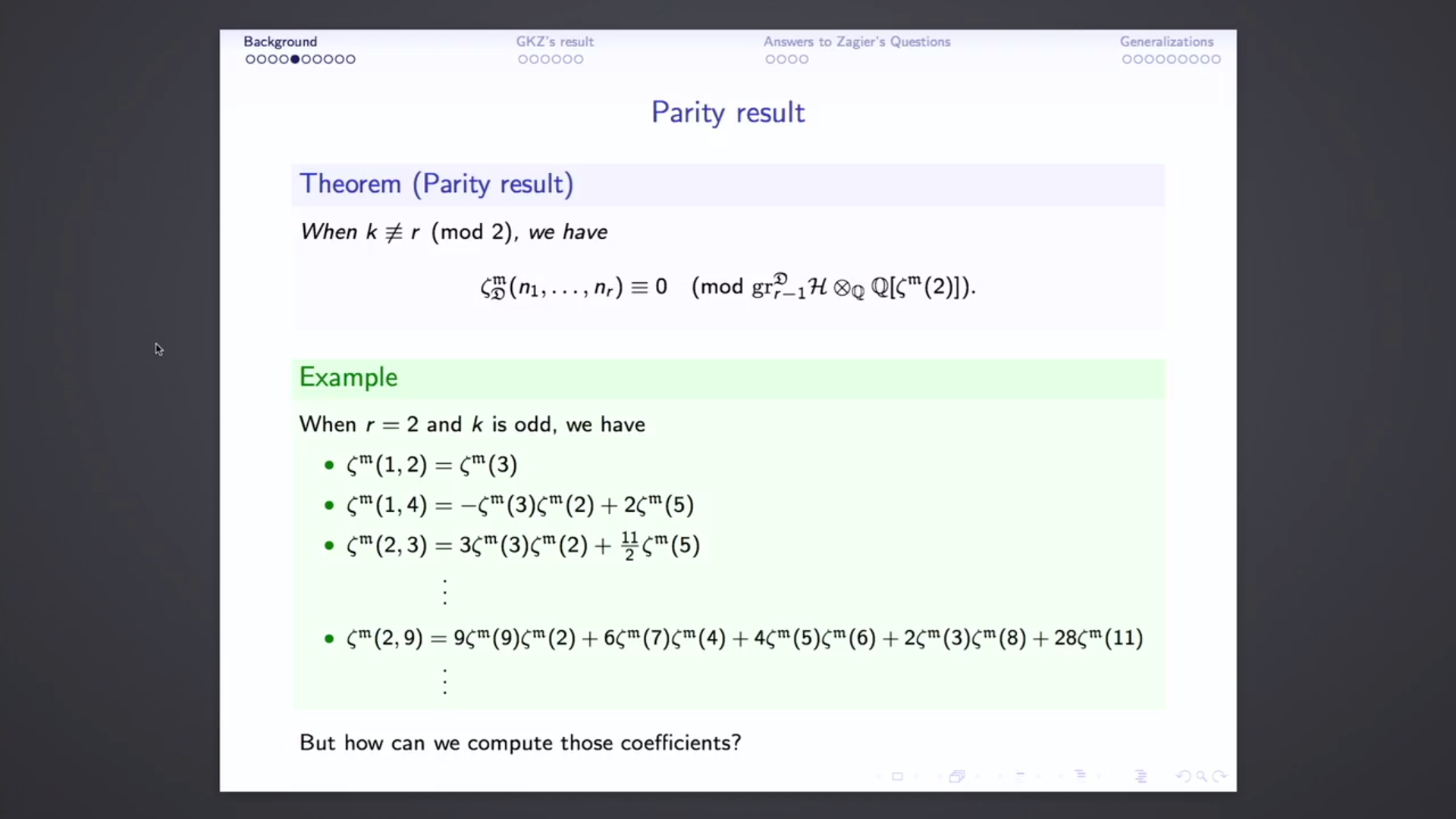
- Zagier formula for double zeta values
- modular forms
- irregular primes
- Bernoulli numbers
- weights of modular forms
MSC:
- 11R34
- 11R32
- 11Rxx
- 11-xx
- 14-xx
- 14Cxx
- 11E45
- 11B68
- 11M35
- 11M41
- 11M06
- 11Mxx
- 14F42
- 11M32
- 11F67
- 11Fxx
Abstract
In this talk, I will introduce the famous result by Gangl-Kaneko-Zagier about a family of period polynomial relations among double zeta value of even weight. Then I will generalize their result in various ways, from which we can see the appearance of periods of newforms in low levels. At the end, I will give a generalization of the Eichler-Shimura-Manin correspondence to the case of the space of newforms of level 2 and 3 and a certain period polynomial space.