Introduction to Ratner's Theorems on Unipotent Flows
Presenter
February 2, 2015
Keywords:
- quasi-immersed manifolds
- dense embedding
- Ratner's theorem
- discrete group actions
- Riemannian geometry
- actions on Lie groups
MSC:
- 53C42
- 53Cxx
- 53C35
- 53C30
- 22D40
- 37-XX
Abstract
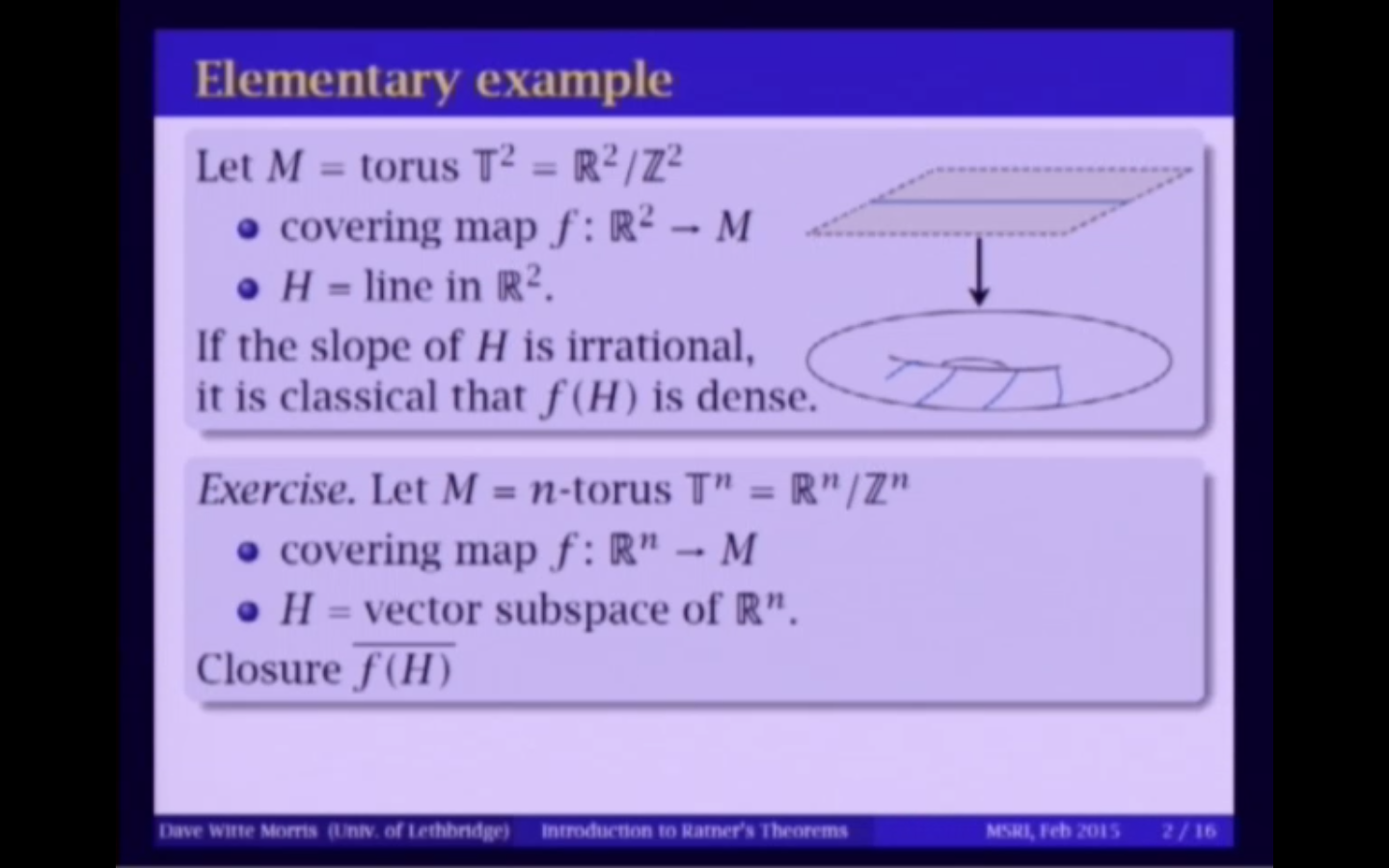
Let f be the obvious covering map from Euclidean n-space to the n-torus. It is well known that if L is any straight line in n-space, then the closure of f(L) is a very nice submanifold of the n-torus. In 1990, Marina Ratner proved a beautiful generalization of this observation that replaces Euclidean space with any Lie group G, and allows L to be any subgroup of G that is ``unipotent.'' We will discuss the statement of this theorem and related results, some of the ideas in the proofs, and a few of the important consequences.