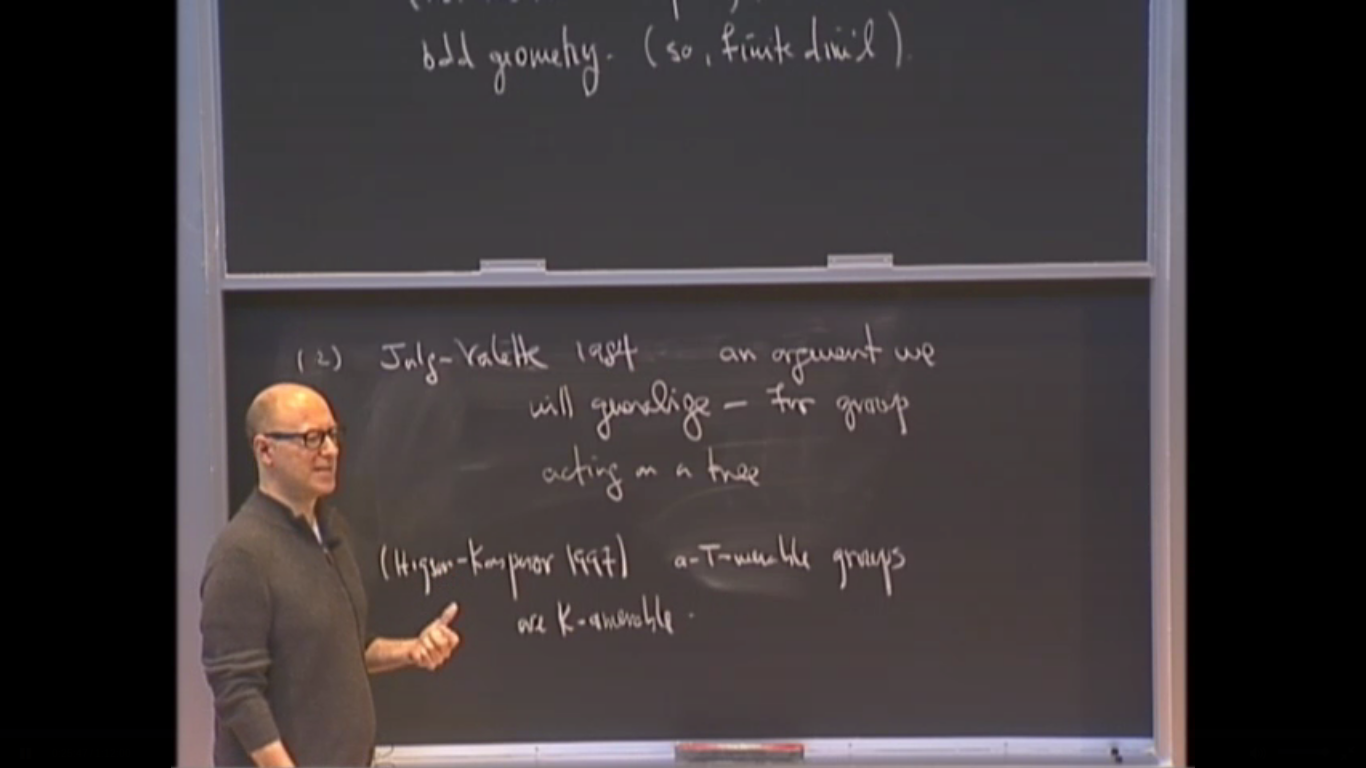Weak forms of amenability for CAT(0) cubical groups
Presenter
December 6, 2016
Keywords:
- CAT(0)
- cube complex
- k-amenability
- amenability
- a-T-menability
- hyperbolic groups
- Cayley graphs
- fixed point properties
- geometric group theory
- Banach space
- group cohomology
- index theory
- expander graph
- non-commutative geometry
MSC:
- 46L80
- 20J06
- 43A07
- 20F65
- 19-xx
- 20-xx
- 43-xx
- 46-xx
- 57-xx
- 58-xx
Abstract
A group which act properly on a CAT(0) cubical complex, while not necessarily amenable, satisfies several weak forms of amenability: such a group is a-T-menable, weakly amenable and K-theoretically amenable. In the talk, based on joint work with J. Brodzki and N. Higson, I will describe a proof of K-amenability which finds its roots in earlier work of P. Julg and A. Valette on groups acting on trees. I will focus on the geometric constructions involved, and will keep the analytic complications to a minimum.