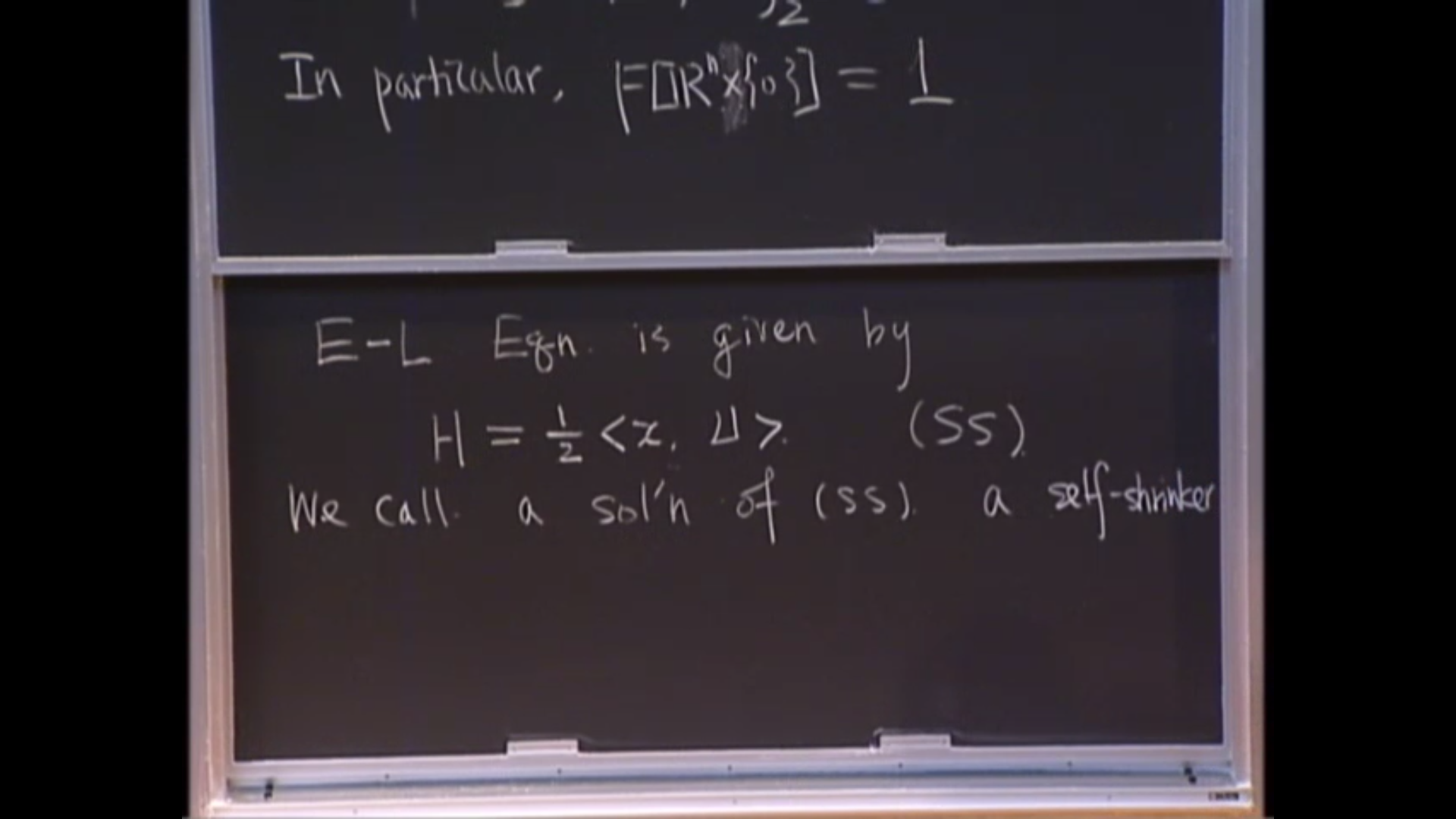The entropy is a natural geometric functional introduced by Colding-Minicozzi to study the singularities of mean curvature flow, and it roughly measures the complexity of a hypersurface of Euclidean space. In this talk, I will survey some recent progress with Jacob Bernstein on understanding the geometry and topology of hypersurfaces with low entropy.