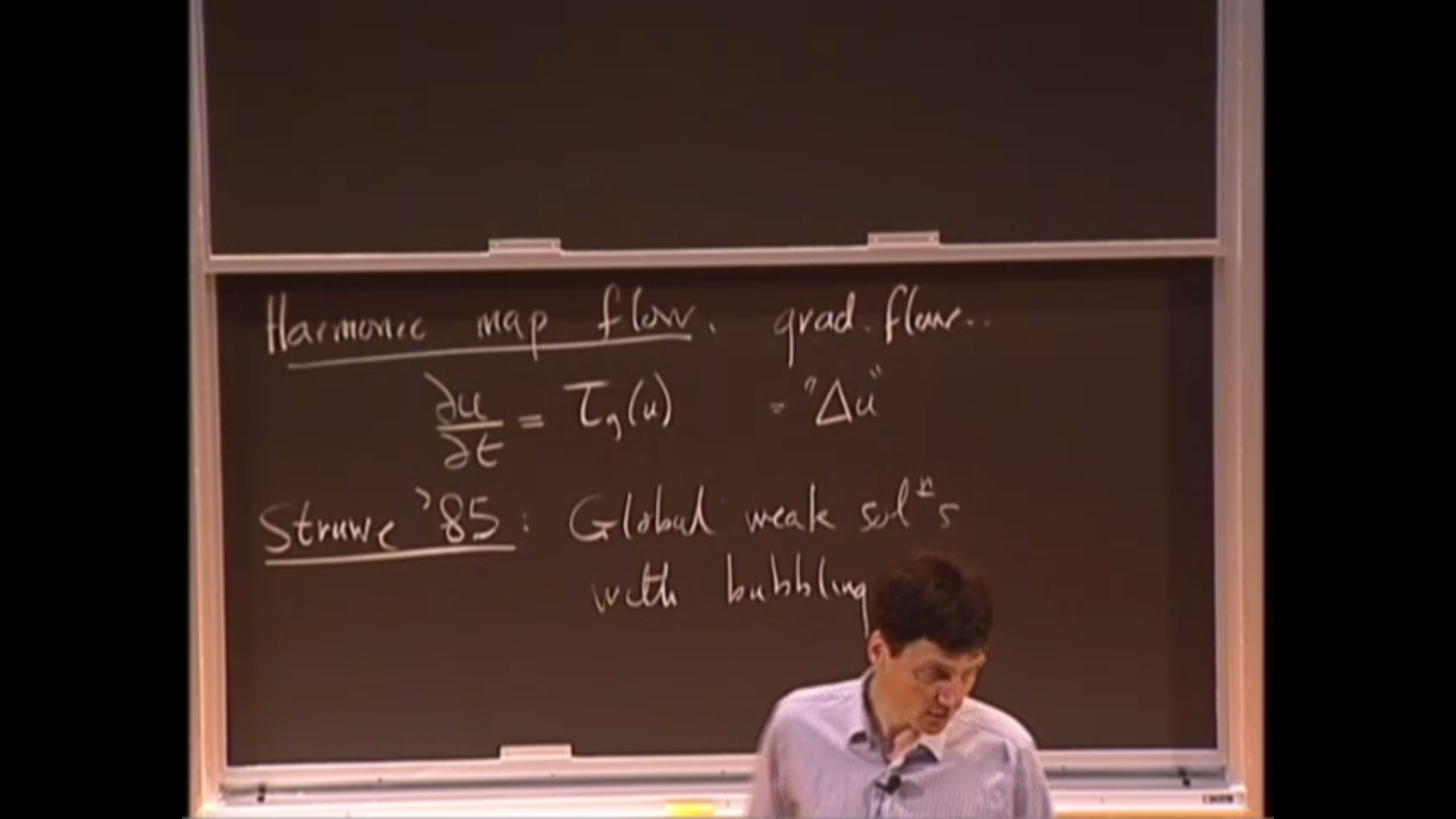The Teichmueller harmonic map flow is a gradient flow of the classical harmonic map energy, in which both a map from a surface and the metric on that surface are allowed to evolve. In principle, the flow wants to find minimal immersions. However, in general, the domain metric might degenerate in finite time. In this talk we show how to flow beyond finite time singularities, and this allows us to decompose a general map into a collection of minimal immersions. This is forthcoming work joint with Melanie Rupflin.