In inverse problems one probes an object with a particular type of field and measures the response. From these measurements one aims to determine the object’s properties or geometrical structure. Typically, the interaction is restricted to a bounded domain with boundary: a part of the human body, the solid earth, the atmosphere, an airplane, etc. Experiments can be carried out on the boundary, and the goal is thus to infer information on the domain’s interior from the boundary measurements.

The mathematical techniques needed to study inverse problems are diverse, and include ideas from the analysis of partial differential equations, microlocal analysis, abstract and applied harmonic analysis, complex analysis, integral geometry, differential geometry, algebraic geometry, control theory, optimization, stochastic analysis, and discrete mathematics. All of these played a part in MSRI’s Fall 2010 scientific program on Inverse Problems and Applications .

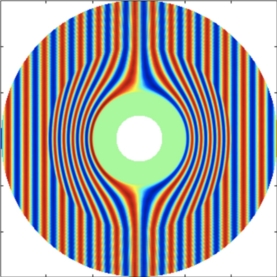Intriguing connections have been made between inverse problems and invisibility—a subject of human fascination for millennia, from the Greek legend of Perseus versus Medusa to the more recent The Invisible Man and Harry Potter. Since 2005 there has been a wave of serious theoretical proposals in the physics literature for cloaking devices—structures that would not only render an object invisible to light rays, but also undetectable to finite-frequency electromagnetic waves. A proposal that has received particular attention, because in principle it can cloak any object of any shape and size, is that of Pendry and coworkers. It has been referred to in the physics literature as transformation optics. Essentially the same idea was formulated earlier in electrostatics terms: a singular transformation is used to blow up a point to a sphere, forming the boundary of the cloaked region. Pushing forward a constant conductivity using this transformation gives an anisotropic conductivity, whose currents have the behavior illustrated in the figure to the right. No current flows in the (inner) ball of radius 1, making this region effectively invisible to boundary measurements. All the electrostatics measurements made on the boundary of the ball of radius 2 are the same as in the case of homogeneous conductivity.

Other constructions using transformations have been proposed; we mention electromagnetic wormholes. The idea is to trick electromagnetic waves to think they are going through a handle. Using an electromagnetic wormhole one can create a secret connection between two points in space. 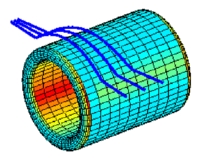One knows the ‘input’ and can encode the ‘output’. The wormhole itself is invisible.

The blueprint of electromagnetic parameters used for cloaking has not been found in nature. Indeed, there is a very active area of research in metamaterials to construct cloaking devices. In a widely reported experiment this was accomplished at microwave frequencies. As stated in Science, the theoretical ideas for cloaking based on mathematical transformations have produced and will produce a long shadow.

A. Greenleaf, M. Lassas and G. Uhlmann, On nonuniqueness for Calderón’s inverse problem, Math. Res. Lett. 10 (2003), no. 5–6, 685–693.

U. Leonhardt, Optical conformal mapping, Science 312 (2006), no 5781, 1777–1780.

J. B. Pendry, D. Schurig and D. R. Smith, Controlling electromagnetic fields, Science 312 (2006), no. 5781, 1780–1782.

D. Schurig, J. Mock, B. Justice, S. Cummer, J. Pendry, A. Starr and D. Smith, Metamaterial electromagnetic cloak at microwave frequencies, Science 314 (2006), no. 5801, 977–980.