During the spring semester of 2013 , the Institute for Computational and Experimental Mathematics hosted a special program devoted to common themes in automorphic forms, combinatorial representation theory and multiple Dirichlet series. A particular focus was placed on computer exploration and the joint development of the required computational tools within the open source mathematical system Sage.

L-functions are vast generalizations of the Riemann zeta function. Just as the zeta function ties together all the positive integers as the coefficients of a Dirichlet series, more general L-series collect different pieces of information, such as Fourier coefficients of automorphic forms, in a Dirichlet series. Sometimes automorphic forms themselves can be naturally grouped into families, and in this case the corresponding L-series can be similarly grouped. In the 1980’s the idea emerged that one could study such families of L-series by constructing higher order Dirichlet series, whose coefficients were themselves different Dirichlet series corresponding to a family of automorphic forms. Such objects were called multiple Dirichlet series. A single L-function associated to an automorphic form will generally satisfy a functional equation, and the multiple Dirichlet series were found to satisfy finite groups of functional equations. The existence of this group of functional equations proved to be a valuable tool for studying the average behavior of the collection of automorphic forms in the associated family.

It was discovered at about the same time, that certain examples of multiple Dirichlet series could be realized as Fourier coefficients of Eisenstein series on covers of algebraic groups. It was further conjectured that every such example had a similar realization. One long term goal of the semester is that the study of Eisenstein series associated with affine Kac-Moody groups may lead to a way to understand the properties of multiple Dirichlet series with infinite groups of functional equations. Such a connection could have profound applications to number theory. The computation of Fourier coefficients of such Eisenstein series amounts to a calculation of certain generalized Whittaker functions associated to representations of a p-adic group. These should be thought of as p-adic “special functions” — the non-archimedean counterparts of famous real and complex-valued special functions like the Gamma function or certain hypergeometric functions. Examples also include some of the most important classes of symmetric functions such as Schur functions and Hall-Littlewood polynomials, as well as Demazure characters.

The discovery that the p-adic analysis of multiple Dirichlet series has striking connections with the representation theory of p-adic groups was only made a few years ago. Therefore, this semester was a remarkable opportunity for researchers in automorphic forms and multiple Dirichlet series to share tools and inspiration with those studying combinatorial representation theory, in particular Hecke algebras, path models, and crystals. In fact, a major impetus for the semester came from collaborations by organizers from separate branches of mathematics on joint computational tools developed using Sage.

The semester featured regular tutorials engineered for students and non-experts in each of the three main components of the semester. Three week-long workshops were held — one devoted to learning about and contributing to Sage, another on Schubert calculus, crystals, and their connections to Whittaker functions, and a final workshop on recent developments related to multiple Dirichlet series. A semester-long course was offered on Eisenstein series for affine Kac-Moody groups.

A major goal of the semester was a better understanding of the ways in which matrix coefficients for p-adic groups and their covers, that is, generalizations of Whittaker functions, were connected to the combinatorics of special functions. This was accomplished by joining the expertise of many of the participants. For example, an improved understanding of the link between matrix coefficients and Hecke algebras, led during the semester to a connection between Whittaker functions and a limit of non-symmetric Macdonald polynomials. The most refined version of the resulting theorem was discovered with the help of computer exploration, and a crucial step was the implementation of non-symmetric Macdonald polynomials in their full generality, which was done with the help of many of the participants.

Previously unknown connections between specialized non-symmetric Macdonald polynomials and affine Demazure characters were revealed by extensive Sage computations, successively peeling off connected components in crystals. General methods for computing these matrix coefficients were also developed and better understood in the context of Ram’s alcove path model.

During the semester many participants, and in particular graduate students, were trained on computational methods and software development methodology. It resulted in dozens of contributions to Sage (>44!). We highlight two team efforts that were made possible by the program. During the Sage Days in February, a group came together to program Knutson-Tao puzzles in Sage.

These are combinatorial objects that describe Littlewood-Richardson coefficients and structure coefficients in various settings. Since many of them are still conjectural (not all of them!), it is a great tool to have to play with and has been used to test various conjectures. The puzzle code now makes the computation of equivariant quantum Littlewood-Richardson coefficients incredibly efficient.

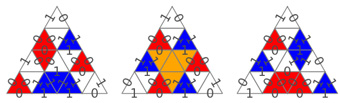
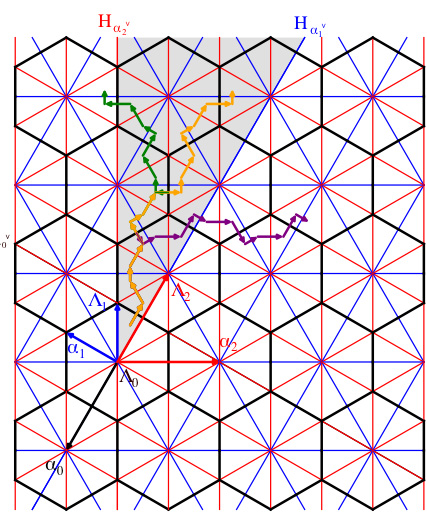
A team of students, postdocs, and researchers made advances on the implementation of crystals and root systems. In particular, support for infinite dimensional crystals is now in place as is the visualization of alcove paths.

Additionally, the program resulted in new introductory material such as tutorials for future users.