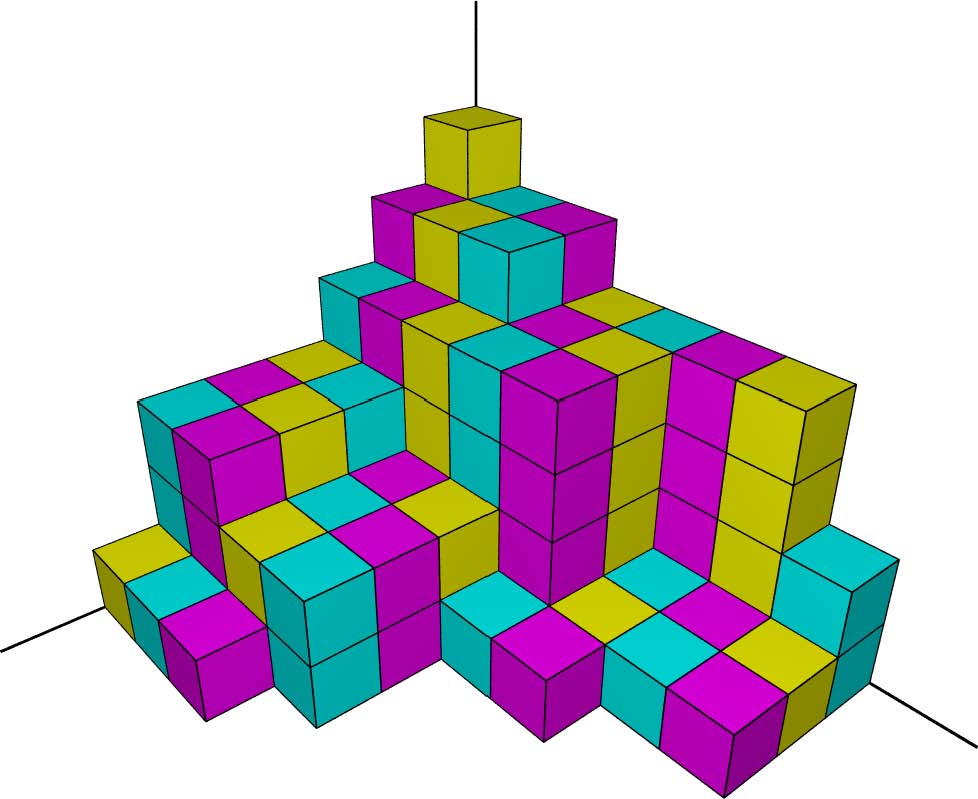How can piles of boxes help us understand the structure of space-time? Algebraic geometry—the subject of MSRI’s Spring 2009 scientific program—provides the link, through the notions of moduli spaces and Donaldson-Thomas invariants .

String theory predicts that space-time is 10-dimensional: Four of the dimensions are the usual three of space and one of time, and the remaining six are curled up into what are known as Calabi-Yau threefolds. These threefolds are what mathematicians call varieties—generalizations of the familiar objects like lines and circles which are determined by algebraic expressions, the same way a line is determined by an equation of the form y = mx + b .

Moduli spaces are used to study and describe families of varieties by parametrizing them, the same way the lines in the plane are determined by different values of m and b in the equation above. Studying moduli spaces associated to Calabi-Yau threefolds will help us understand them and hence understand the implications of string theory. One especially important moduli space consists of ‘stability conditions’ associated to a Calabi-Yau threefold. To understand this space, one tries to ‘count’ stable objects and to determine how the count changes as the stability condition varies. This leads to the sophisticated mathematical notion of Donaldson-Thomas invariants , but remarkably, these invariants can sometimes be computed by concrete, combinatorial means: Namely, by counting the number of ways n boxes can be stably stacked in the corner of a room. Conveniently, these piles of boxes, or 3D partitions , have been around since at least 1916, when it was proved that the number of 3D partitions with n boxes is precisely the coefficient of q ⁿ in the expression

∞

∏ ( 1 – q ^m ) ^-m

m=1

Algebraic Geometry has grown dramatically over the past century, with new subfields constantly branching off. The above discussion necessarily represents only a fraction of the recent progress in the field, but it does reflect some general features of algebraic geometry—mixing the classical and the modern, and continually finding surprising new connections to other parts of mathematics. MSRI’s Spring 2009 program on algebraic geometry featured numerous workshops, each of which focused on a different aspect of this broad subject while involving and uniting mathematicians from diverse backgrounds.

K. Behrend and B. Fantechi, Symmetric obstruction theories and Hilbert schemes of points on threefolds, Algebra & Number Theory 2 (2008), 313–345.

M. Gross, D. Huybrechts and D. Joyce, Calabi-Yau manifolds and related geometries, Springer, Berlin, 2003.